What Modeling the Pandemic reveals about our Mathematics

Authors : Arul & Tathagata

Ever since the COVID19 pandemic took over the world, we have seen an explosion in the scramble among the professional scientific community in trying to make sense of the disease. As of 4th April, aRxiv, medRxiv and bioRxiv lists a combined number of 1424 articles (278+886+260) on COVID19, within a span of around 2 months. The mathematicians of the world in particular have been busy mapping the spread of the virus. Both the science journals and popular media spaces are abuzz with discussions about modeling the epidemic, and simulating its spread. The Indian scientific community is no exception to this. In this essay we try to look at the modeling efforts going on in the mathematics community – across the countries and specifically in India, and through that raise certain critical questions about the nature of Professional Mathematics, and its relation to Society.

Mathematics is Apolitical!

Mathematics is consistently touted as an ‘apolitical’ knowledge system. It is seen variously as a ‘language’, a ‘grammar’, a ‘logic machine’, and such. All of these descriptions invariably lead to the conclusion that mathematics is devoid of politics, that it is objective and value free. The growing incidences of machine-driven theorem proving, presently ongoing works in the field of machine-learning driven mathematics research, etc., are seen as leaving little doubt about these claims. Ironically, the same machine-driven mathematics research has also brought another kind of question to the fore – those regarding the agency of the human mathematician. What is really the role of the human mathematician? Assuming in near (or not so near) future bulk of mathematics research will be taken over by intelligent machines, would we still need human mathematicians?

The tentative working answer to this question, at least as of now, is that we would still need the human mathematician to pose mathematical questions – which may include even questions coming from ‘out of the blue’. The human mathematician still retains the agency of posing a mathematics question. Once a question is posed in proper mathematical terms, arguably the objective, value free theoretical machine of mathematics takes over, and if proper tools are chosen, in the proper direction, then one is led to the answers by mathematics itself, without any scope left for any further subjective intervention by the flawed human agent. It is precisely at this point that we draw attention to the mathematics of modeling a pandemic.

Modeling a Pandemic

There are numerous simulations that are making the rounds, trying to explain the spread of a pandemic. Such as this one from the WashingtonPost:

Awareness building popular science materials such as videos curated by TIFR scientists, are based on similar simulations. Most of these simulations are based on the so called SIR (Susceptible, Infected, Recovered) model in epidemiology. These models categorise the population into three – susceptible (those who are not yet infected and hence susceptible to infection), infected and recovered. The basic assumption of the model is that whenever a susceptible person comes in contact with an infected person, they get infected.

In their popular appearances most of these model-based descriptions start with a kind of translation from the ‘human’ to the ‘mathematical’, such as this paragraph from the WashingtonPost report – “We will call our fake disease simulitis. It spreads even more easily than covid-19: whenever a [blue]• healthy person comes into contact with a [brown]• sick person, the healthy person becomes sick, too.”

Models come with caveats that mention that they are over-simplified, and that the real situation is more ‘complex’. But at the same time the very act of modelling is about throwing away complexity and making the modeled as simple as possible.  For instance, the WP article mentions that, “In real life, of course, people eventually recover. A recovered person can neither transmit simulitis to a healthy person nor become sick again after coming in contact with a sick person.”

The question however is, what is the extent of this ‘complexity’, and what kind of complexity do we as mathematicians choose to acknowledge in our models, or even as extrinsic to our models, and what kind of complexities do we choose to ignore altogether and what does this ignorance lead to. After all dots are dots, and humans are humans.

Before getting into this we discuss a model of COVID19 spread in India.


Of the many modelling exercises related to COVID19 from India, the one to catch widespread attention was a preprint titled “Age-structured impact of social distancing on the COVID-19 epidemic in India”. This research incorporates information about population distribution across age groups and social contact structure (the degree of interaction between different age groups) into the traditional SIR model.  The main job of epidemiology models is to predict the spread of infection as a function of time. Given that the risk factor for the COVID19 varies across age groups, incorporation of age as a variable can make the prediction of epidemiology models of COVID 19 more robust. Since this model incorporates social contact information it can not only predict the spread of infection but also the effects of a lockdown. After all, from this model’s perspective, all which a lockdown does is eliminate most forms of ‘social contact’. The model (and thereby the mathematicians who developed the model) suggested that a 21 day lockdown is insufficient to control the pandemic in India and demonstrated different possibilities for a lockdown, with a 49 day continuous lockdown being the best of them all (the below panel corresponds to these predictions by the model. A copy of the panel can be found in the preprint, the copy below is taken from social media). This conclusion and the figure below was carried by multiple mainstream news agencies (1, 2). In addition to that it also found its way into social media and had become the WhatsApp status of many. All this was happening while lakhs of displaced workers were walking hundreds of Kilometers to go home as the lockdown had abruptly disrupted their lives.

The popularity of the model was despite a very visible flaw. In the figure one may see a sharp fall in the number of infected from the moment the lock down is imposed – this is impossible since we have constantly been hearing that the symptoms of COVID appears between 2nd and 14th day of infection, so at least for 14 days after the lockdown the number infected cannot fall so fast.  This is essentially due to two factors,

1. The model assumes that there are no asymptomatic individuals in the population at the time of lockdown, that is the model assumes that everyone who is infected till the day lockdown was announced is identified and removed from the non infected population.

2. The model assumes a perfect lockdown, that is it assumes that lockdown eliminates all contacts outside home.

In addition to this it also deems the pandemic to be under control only if the number of infected is below 10, an arbitrary choice of number which is not backed by any data or theory. In fact the choice of 10 as a lower limit for a country of 1.3 billion is rather surprising.  While these are mathematically valid assumptions (you may make any assumption to set up a model), they lead to extremely erroneous real world conclusions. For instance see below how the predictions of the model compares with the number of infections after the lockdown was implemented. Not only that there is no sharp fall, but the number has been increasing since.

While one may argue that the rise in numbers is due to various factors, assumption 1) is clearly epidemiologically invalid for COVID 19, while assumption 2) is socially invalid (it is impossible for people to maintain physical distance in areas like Dharavi where families may have to share toilets). Thus we have a model which is mathematically valid but is of little use in tackling the pandemic. This raises two questions, what is it useful for then? And why do such models get produced? The answer to the first question is that it gives a way for other mathematicians to incorporate age structure and social contact structure into their models. The answer to the second question is closely tied to the act of modelling itself. As mentioned earlier the main objective of modelling is to reduce complexity and make predictions. This choice of reducing complexity is, in mathematical terms, the choice of the mathematical question that the human mathematician chooses to pose and engage with.

Not just a Social choice, but also Mathematical

Let us take a step back, into the world of mathematics itself, and see how this is not just a social-political concern, but in fact a mathematical concern as well. Let us take for example the case of the so-called ‘stereographic projection’.

The stereographic projection is essentially a mapping of the sphere minus the North Pole onto the flat plane, and can be thought of as placing a point light source at the missing North Pole, and looking at the shadow cast by each point of the sphere onto the flat plane underneath. This map works in situations where we are mainly concerned with one-to-one continuous mappings between points on the (punctured) sphere and the flat plane. But as differential geometers have established, there can not in fact be any ‘reasonable’ mapping between (even a part of) the sphere and a flat plane that preserves distance. This is why no matter how we map it, the world map on a flat surface always distorts distances.

Therefore, though choosing the stereographic map might serve useful mathematical purposes in certain contexts, it may be quite useless in various other kinds of questions. Whether we use this set-up or not, therefore, is solely dependent on the kind of question we are dealing with.

Is our Society a Uniform Distribution of freely mobile dots?

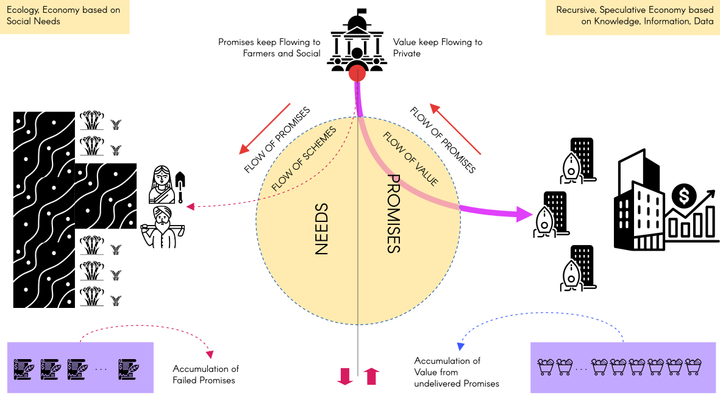
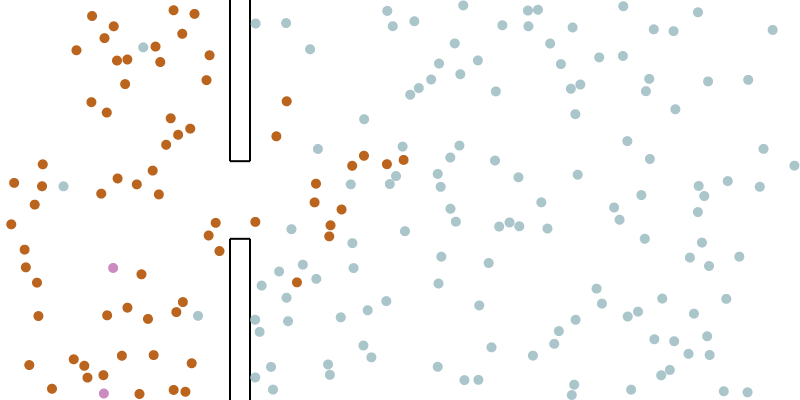
In our pandemic simulation models, beginning from the popular press articles, to scientific journal publications [1,2], most often we use a uniformly distributed model of human society, with freely moving dots representing human bodies. But human society is not so uniformly distributed. In a recently published essay titled “Germs, Stains and Our Pure Society”, author V. Geetha argues, “Without disrespecting the efforts of all involved in warning us of what might yet transpire, it yet appears shocking that the value of life has not to do with the richness of existence, but of sheer survival. For the worker, though, it is clear: should they choose death through hunger or death through illness? Such clarity is an indictment of the political and social order, of all of us.”Human society, including the Indian version, is graded by all kinds of hierarchies – both social and economic. And there is no way to represent such a hierarchical grading in the form of a uniform distribution. What should have been a natural premise for beginning any simulation process is therefore a segregated model. Indian society is segregated into tight separate compartments according to caste, which – barring a thin spectrum of exceptions perhaps – is also coincidental with the class divisions. Let us therefore think of a model where instead of one uniform region of dots, we have 2 separate regions, separated by an impermeable barrier. Indian society, not unlike the rest of the global human societies today, is extremely unequal. According to this report, “India’s top 1% bag 73% of the country’s wealth”. Let us say, the physical space in our simulation represents not the ‘physical space’ in real terms, but the ‘space of opportunities’ that exist. In this specific situation of a country under complete lockdown because of the pandemic, this might mean who gets to order ‘contactless’ food online while being ‘quarantined’ at home, and who doesn’t. In our segregated simulation space therefore, the top chamber must occupy 73% of the total space, and the bottom chamber 27%. Also, 1% of the total number of dots occupy the airy top chamber, while the remaining 99% of the dots occupy the bottom chamber. It would look something like this:

Suddenly something that so far looked more like a Brownian motion modeling problem, begins to look more like a sphere packing problem.

Of course, in this case, the space enclosed in the chamber is purely representative of wealth thought of as ‘space’ in the sense mentioned above. The real life situation will also have to factor in real physical space. In other words, the real life models would be an ‘amalgamation’ of sorts between this kind of a situation, the WP kind of situation, and possibly other such factors.

The point is however, a simulation or modeling that pays no attention to the class, caste and other separations and hierarchies that exist in society, and pushes such concerns away citing the need for mathematical practicality, is not a practical model – mathematically, as well as socially. Just like the stereographic projection is mathematically impractical for questions that require distance preserving maps.

And it is precisely this ‘setting aside’ of certain key premises, where the use of mathematics – when confronted with social realities – becomes deeply political. Once the mathematician has successfully set aside a certain premise, the rest of the simulation or modeling or optimization program is purely mechanistic, objective, value free, and so on.

Mathematics as a mask

This ‘objectivity’ soon becomes a convenient and fancy mask for protecting ourselves from the dust storm of lost premises. And therefore the politics of it. This hidden politics can of course have serious implications, specifically when the question is about millions of lives at risk.

If the model we discussed earlier had remained in academic circles it would have been a ‘novel’ academic contribution (for bringing in age structure and social contact structure for the first time in SIR models in Indian context) with some faulty assumptions, which either the authors may have been asked to fix during review or others might have worked on it and improved it. An obvious direction for further research then would be to drop assumption (1) and use the SEIR model of epidemiology – SEIR models have one more category, E for exposed (in simple terms this category can account for asymptomatic individuals).  The author(s) here were witness to a discussion among a group of scientists and mathematicians on the model.  The general responses were mostly along those lines mentioned earlier in this passage, with some exceptions. The verdict was that it is a ‘nice paper’, with ‘good ideas’ but with an ‘inappropriate model’. While this was happening on the one side the predictions of this inappropriate model were being widely shared among people. The paper may not be considered by the policy makers while deciding on a post lockdown plan, but if they do the mathematically valid but ‘inappropriate’ model will affect the lives of millions. Incidentally the group where such an opinion was being expressed also happened to be a group which is involved in busting hoaxes, and they thought a paper which is mathematically valid but not practically useful (possibly socially damaging) need not have a public scrutiny/debunking but only a ‘peer review’. This, one may read as symptomatic of the academic community itself, which is inward looking and is unconcerned about the potential damage their constructs can produce. The accountability is turned inwards and is reduced to the process of peer review. The outward facing activity is limited to science outreach and popularization and ‘hoax busting’.  A deeper analysis of this phenomenon will require characterization of academia and it’s epistemologies (it’s questions and methods) along the lines of class and caste interests. Such an analysis is beyond the scope of this article. Here we get back to the social implications of such modelling practices.

The False Destinations

All of these models talk about the need for ‘social distancing’ as a necessary step towards reducing the spread of the infection. This is essential in order to release the pressure on an already stretched health infrastructure. Slower pace of the spread of infection also allows the Government the time and resources necessary for setting up emergency health infrastructure that is crucial in times of pandemics.

In its simulations, the WP article denotes social distancing by the healthy blue dots going stationary. What it means by that is basically the curtailing of actual physical mingling of the dots – the people. It does not mean the stopping of physical mingling of people with consumption goods, or with essential service consumptions. Of course all such things are necessary for the dots to survive in the first place. Thus although they might look stationary, they are actually virtually mobile. We could think of this as a necessary ‘buzz’ in each dot.

Clearly, such a fuzzy ‘buzz’ requires enough degrees of freedom around it – that is, space. As is clear in our situation of the lower chamber, no such degrees of freedom are essentially available. In most of the pandemic simulations, ‘death’ occurs only through contracting the disease. But let’s say in our case, death was as likely, if not more, if the buzz were to be frozen. Then it becomes a whole different modeling question altogether – how do you physically distance, while also maintaining the buzz for the people. Mere ‘social distancing’ would no longer be an answer to such a modeling question.

Exactly this we are seeing currently in this country, after the Government announced a nationwide lockdown. The decision for such a lockdown was taken purely based on the constantly repeated mantra of ‘social distancing’ in popular media and scientific jargons. No prior preparations were made to ensure living conditions for the crores of workers, mostly working in the unorganised sectors without any kind of social security, most of whom are displaced workers working far away from their homes, in essentially foreign lands.

And we saw the fallout. People did their own optimizations at individual and community levels. They decided that while contracting the disease is still a game of chance (as, incidentally, the pandemic models would also agree), possible death from hunger or inaccessibility of essential services alone, was a much more likely outcome.

So they decided to start back for their homes. For days, before the state boundaries were sealed off, we saw an unprecedented scene of lakhs of displaced workers walking along the length and breadth of the entire country, across hundreds of kilometers on foot, for days together. If the official death toll from COVID19 was in the early 30s from the first death weeks ago, the official death toll of workers dying from sheer exhaustion of the walk, and from getting crushed under heavy vehicles on highways – was in the late 20s within just one day.

But since the pandemic modeling was fundamentally flawed, these deaths will never be accounted as ‘COVID19 deaths’. We saw reports of people being forced to eat grass, and of workers committing suicides. None of this was accounted as social fallouts of the modeling of the epidemic. None of this had any space or time for mathematics as we know it. Right now as we are writing this essay, there are entire slum rehabilitation buildings under lockdown in Mumbai, where COVID19 positive cases were found. The residents, even if they are still not infected, have been screened once and forced to stay inside their rooms. Police have been posted right outside to prevent anyone from stepping out. One elderly person reportedly died in one of those flats recently, allegedly because his family members were prevented from getting medicine supplies.  Such moves have no justification whatsoever, even if we were to take the model-dictated ‘social distancing’ seriously. But such false outcomes are inevitable, since the model itself is fundamentally flawed to begin with and is bound to throw up such contradictions. One could discuss several such aspects of flawed implications flowing out of such mathematical modeling, but we only point out one more here. While discussing the need for strict quarantine, the WP report says, “To slow simulitis, let’s try to create a forced quarantine, such as the one the Chinese government imposed on Hubei province, covid-19’s ground zero.”

Through the above simulation the author then goes on to demonstrate what could happen if the quarantine is not strictly enforced. “Whoops! As health experts would expect, it proved impossible to completely seal off the sick population from the healthy,” it says. Thus the “complete sealing off of the sick population” is presented as a kind of health morality in the context of a pandemic. Anyone breaching this morality is committing nothing less than a crime against humanity. This has serious implications, particularly in a country like India with its brutal history (and present) of the caste system – where sanitation morality has been used for ages to criminalize vast sections of the working population. Here is one example of what this can lead to for people: in a shockingly criminal incident, the UP administration doused displaced workers returning during the lockdown with chemicals meant for cleaning buses. Needless to say, these workers belong to the oppressed castes of Indian society. What is not criminalized in models such as the above is the killing off of the lower chamber people in our model, purely through freezing them to death, if not by forced contamination because of the cramming together.

All too often, the mathematician brushes off such questions as problems of the society, and thus beyond their purview of things or expertise or, at times, even concern. However, like we have tried to argue, our mathematics itself should have factored such things in.

The Missed Destinations

Coming back to our proposed simulation premise [Figure 6], it seems to be the set-up for a (complex) thermodynamic diffusion model. There are two ‘heat chambers’ separated by a non-permeable diaphragm. There is pressure from the below chamber to expand into the upper chamber, but there is energy that is being spent to keep the separation where it is, and if anything, to push it even further down, to further squeeze on the lower chamber. The chief engine running this process is the State machinery. The fuel is nothing but the labour of the people from the lower chamber. The whole setup is what we could call our political economic system.

If this were indeed the case, then any reasonable mathematical model must discuss the ways to break the insulating separation between the two chambers and allow controlled dispersion of the dots – a process that would be very different from what is meant by the catch-phrase of ‘social distancing’. This rupture is crucial for any possibility of physical distancing between the lower chamber dots. It is only after such a rupture that we get back to the situation described in the WP article, and possibly follow the norms it mentions there on.

In case breaking the insulating barrier is not possible, the least that must be done is to reverse the engine driving the system (the State apparatus), so that the barrier gets gradually pushed up till the point that the chambers occupy spaces that are in proportion with the number of dots they are inhabited by. In this case therefore, the separation should be pushed up till the time the lower chamber fills up 73% of the total space.

Such modeling of the situation could be easily done by the mathematicians. Social implications of such a model would be measures such as unconditional debt cancellations of the working population, free and fair ration supplies, rehabilitation of people from crowded urban areas into the lakhs of unsold apartments across Indian cities, State-funded transportation arrangements to ensure displaced workers reach their native villages under proper medical testing, treatment and care, redistribution of the professions ladder – in case of India this should for instance, involve upper caste population being asked to put their own labour for cleaning their own waste, while the traditional sanitation workers are relieved of such tasks finally so that they can take care of their own families and neighborhoods. Implications would also include re-categorization of what is considered high-skilled labour, declaring sanitation and health work as high skilled work and inverting the pay structures accordingly, health insurance and proper benefits for the health and sanitation workers, release of prisoners, aggressive testing and contact tracing that would minimize the needs for a complete lockdown (like for instance South Korea and Taiwan seem to have done, and with exemplary results), mortgage payment cancellations, universal public distribution system, re-defining what is meant by ‘essential services’, and so on. The Indian mathematics community could have routed for all of these measures, ‘mathematically’ and not just socially.

Instead however, ‘Social Distancing’ and ‘Flattening the Curve’ ended up becoming the Ideological State Apparatus for maintenance of the status quo, thanks to the scientific community.

The term ‘flattening the curve’ stands for the idea that the spread of the epidemic should be maintained below a certain horizontal line which represents the maximum capacity of the healthcare system. This is also a question of optimization, optimizing for the limited healthcare resources. The time frozen notion of flattening the curve masks another possible angle of optimization – enhancing the health care facilities. In modelling parlance this would amount to raising the horizontal line. Ideally we would like to optimize from all possible directions, but then we hear only about the flattening of the curve. The question of raising the line is more political than that of flattening the curve, the former amounts to demanding the governments to spend more on health care and enhance their facilities on wartime footing, while the latter is about outsourcing the responsibility on to the people by ‘socially distance themselves’.  And our apolitical mathematics appears to have made a clear political choice of which question to ask which not to ask.

In a way, it can be argued, that the outcome of ‘Social Distancing’ led to the modeling in the first place, rather than the other way around. Once ‘Social Distancing’ becomes the norm, then all that remains is the dry mechanistic mathematics of optimization – how do we optimize our resources and infrastructure mobilization so that ‘minimum damage’ is caused during lockdowns, how long should we impose a lockdown, which of the listed ‘essential services’ should be allowed to run, and so on.

What are we Optimizing?

But what is it that we are actually optimizing? As we have tried to argue above, the actual optimization is taking place in the set of problems posed. Once a problem has been chosen, the optimization process that follows in terms of finding a solution is mostly a decoy. In algebra, one can formally deal with the ‘space of all questions’. As an example, we can on the one hand talk about the set of all polynomial equations (our analogy for ‘questions’), or we can talk about the set of solutions of any particular, or a bunch of, given polynomial equations. What kind of solution sets we get, of course, depends on what set of polynomials we choose to work with.

Just to be clear, even after a set of polynomials is chosen, there are mathematical choices that are to be made, such as, “Which is the universe of solutions we are willing to consider?” For example, the famous equation :

x2+1=0

It has no solution in the world of Real numbers. But as we know from abstract mathematics, mathematicians have found out a way to get around this by declaring a letter (the ‘imaginary number i’, to be precise) as its solution. On the face of it this looks like just a way of shifting the buck. But what this small move does, is to open up an entire new world of mathematics of the Complex numbers.

And in fact, mathematicians have even figured a way to think of the abstract ‘imaginary numbers’ in very real terms - in terms of rotations of the flat plane, where i represents rotation by a right angle (90 degrees). The point is, mathematicians have found out ways to not only ‘cook up’ abstract solutions to questions that were thought to have no solutions in the known established world (the world of Real numbers, in this case), but often such cooked up new worlds have become concrete themselves based on shifts in how mathematicians made sense of them (Rotations of the plane, in the case of imaginary numbers).

Coming back to our issue at hand, once we have chosen the project of optimizing a lockdown, often several such questions concerning what all measures are possible to ensure that we save as many lives as possible - the set of solutions in other words - is itself further optimized, by declaring that certain steps are ‘impossibilities’. Such as, the measure of rehabilitating quarantined slum residents in the lakhs of unsold empty high-rise apartments in a city like Mumbai, or that of diverting the thousands of crores of Rupees from mega-construction projects into health sector on an immediate basis (These and several other measures have been demanded in this Open Letter to the CM of Maharashtra recently by a group of social activists).

The mathematicians for whom almost nothing is ‘impossible’ in the world of abstract mathematics, who can create new worlds - new ontologies - in that abstract Universe at will, almost like Gods themselves, suddenly when it comes down to saving people’s lives during a pandemic, ‘practicality’ questions become optimization constraints, cutting down both the set of questions allowed, and the set of solutions allowed. While rehabilitating homeless people in high end empty flats is seen as impossible or impractical, deaths of scores of displaced workers who are forced to walk for hundreds of kilometers, is on the other hand, quite acceptable in terms of practicality.

This is largely because there is in fact another optimization that is going on behind the scenes, in the unspoken collective mental worlds of these mathematicians - the optimization of the availability of labour force.

Hidden Optimizations

If the workers of this country - particularly those in the ‘essential services’ - were in a political position to go on en masse strike, or if there weren’t a huge standing army of unemployed labour, our mathematical models would have paid the required attention to the modeling-related questions we have tried to raise here.

Our mathematicians, as a professional class, largely homogeneously upper caste in terms of social composition, know that at the end of the day - no matter what policies their models lead to - their own supplies of sanitation workers, health workers, food producers, delivery persons, electricity workers, telecom sector workers, mining sector workers, and so on, are guaranteed. And the fact that they know this, has a direct impact on the models they produce. In other words, the models that are produced are not a product of the mathematical machine, but of the class-caste location of our professional scientific community. Their models can therefore conveniently ignore a situation that looks like [Figure 3], and take a mere slice of the upper chamber before going on to project that as the Universal reality. The mathematics that follows thereafter, is extremely effective in hiding this class-bias of the entire model itself. Like ritual mantras, we keep minting media catch phrases such as “Flatten the Curve”, and producing numbers that project possible deaths, possible infected persons, etc, etc. Numbers and graphs churned out by ‘experts’ are hailed as infallible Gods that are here to rescue us.

But in fact the basic thing that all this is based on, is our mental estimates of the patience of working people of this country - how much further can they be pushed, how much more can we outsource to them the social costs of a pandemic, how much more can we bank upon our coercive social structures to further squeeze them. The day, if at all, this patience falls apart [and it is not clear if there are any attempts to ‘mathematically model’ the patience of our working classes, or if such models will have that kind of stochastic predictive powers], our current modeling of pandemics will be rendered totally meaningless.

While on the one hand we have the official modeling projects, and the hidden ones that are going on in unspoken terms inside our class-caste networks, it is also worthwhile to take stock of what are some of the modeling questions that our mathematicians do not even seem very interested to work on.

What are we not modeling?

Just like we are not modeling the patience of our working classes, there are various other aspects of the pandemic-stricken society that we are not modeling as well. Our mathematicians are not modeling incompetence, arrogance and greed at the highest levels of power, when it comes to dealing with something like this crisis. Our scientific community does ‘hoax busting’, but makes no scientific or mathematical attempt to understand the premium or value that is being put on ignorance and anti-science such as what we have seen in such times. We hardly make any attempts to model the ‘infodemic’ - the spread of misinformation, and agentative fake news - through news and social media alike. This is particularly frustrating because the mathematics of modeling disease spread is very similar to that of understanding spread of rumours. The very simulations that are making rounds in the popular media about the spread of the virus, could have been used to study the spread of false information and fake news. We have no mathematical idea of how the infodemic - particularly the ones targeting specific communities of people as agentative carriers of the virus, as was seen in the case of Muslim worshippers at the Tablighi Jamaat in Nizamuddin, Delhi recently - is compounding the social preacarity of the already vulnerable sections of people. We are not modeling the spread of communal hate, specifically in the pandemic context. We are not modeling the changing labour patterns, credit patterns and so on. We are not modeling the aftermath of the 21-day national lockdown.

Even if some economists and social scientists might be doing some of the above, these projects are not seen as integral to understanding the pandemic itself. After all when a person belonging to a minority community gets lynched, fueled by the fake news campaigns, such deaths will not make it to the list of ‘COVID19’ deaths just like the displaced worker deaths also did not.

There is no denying that Mathematical models have contributed to effective tackling of crisis situations in the past, and they certainly are giving insights into the spread of COVID19 pandemic as well. But that is all they are, tools to give some insights (into the future) about the behaviour of a phenomenon. As we indicated earlier they exclude/ignore more than what they include. They can at the best inform policies but cannot really become the basis for policies. Policies affect people and even a single misstep can have damaging consequences, whereas Mathematical models if ‘inappropriate’ will only have to be revised. Mathematical modeling, and perhaps our Mathematics itself, might look very different if they were generated by Mathematicians from different socio-economic locations than what we have at present. For example, if one of the lower chamber ‘dots’ had to model the situation, and prescribe policies, we would perhaps have a very different approach to the task of modeling.

As a people, we must inculcate the habit of being skeptical of public policy hiding behind the power of numbers and graphs. A seemingly ‘mathematical’ argument, or something from an ‘expert’s’ desk, is not necessarily valid. Today, we have pseudo-science and anti-scientific hoaxes on one hand, and we have ‘scientific hoaxes’ on the other. The only way to bust hoaxes from our so-called scientific community is for the common people to ask them: What they are modeling? What are they not modeling? Which questions are they asking?  As scientists or mathematicians, what questions are they avoiding? What biases are they hiding under the carpet of their mathematics? What is at stake for this class of people?

Here is what a philosopher from the ancient times had to say about ‘criticality’:

Do not believe in hearsay; Do not believe in what is handed down through generations; Do not believe in anything because it is accepted by many; Do not believe because some revered sage or elder makes a statement; Do not believe in truths to which you have become attached by habit; Do not believe merely on the authority of your traditional teachings.

Have deliberation and analyse, and when the result accords with reason and conduces to the good of one and all, accept it and live up to it.”The Buddha, The Anguttara Nikaya, I, 188.

Today, after 2500 years, this would be a good time for us to take lessons from this, as a society. There is no short-cut to this. Developing such a culture of skepticism is a long drawn social process. But there is no alternative to this. Because the oppressed, marginalised, working people of this country are on their own. And facing an epidemic - not just of a virus, but also of ideas.